If Θ is an angle made by connecting (0,0), (1,0) and any other point on the unit circle M = (a,b), then cosΘ = a, sinΘ = b, or equivalently: (a,b) = (cosΘ, sinΘ)įor example: the line for Θ=p/4 radians crosses the circle at (sqrt(2)/2, sqrt(2)/2). The unit circle below gives the commonly used angles and their sines/cosines. (For this article, p will denote pi=3.14159…)Ġ degrees = 0 radians = 2p radians = 360 degrees (Note that 0 and 2p are often used interchangeably) The unit circle is segmented into 360 degrees or 2*pi radians. Until now, you probably measured angles in degrees. It is also helpful as a handy homework reference.
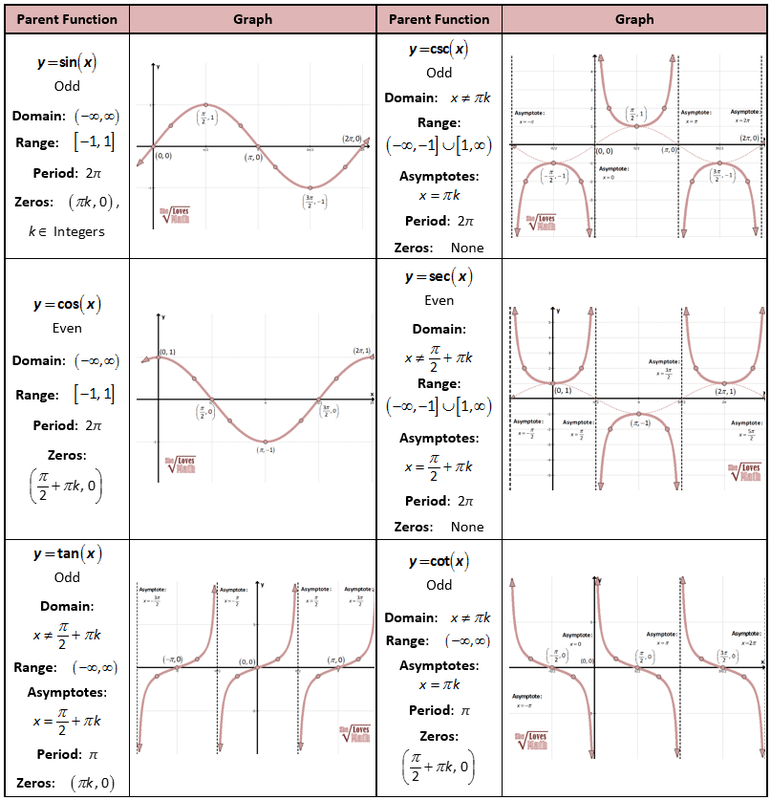
For those of you that have studied the material before, this is a condensed formula sheet to help you prepare for exams. If you don’t have any experience with the subject, there is not enough information here to teach it to you.
Before you start reading, you should know that this is only a review.